What @Burningstone and @PhilMongoose say.
Stab again at the Modern Portfolio Theory (MPT) and/or the Sharpe ratio, @Julianek, but instead of using your dagger I would recommend taking a swing with a two handed sword like this one:
Even the first time I read the reference text on MPT (for me the 9th edition of Modern Portfolio Theory and Investment Analysis by Elton, Gruber, Brown & Goetzmann, e.g. see here), my immediate reaction was that they are just using volatility as a measure of risk simply because you can calculate it (using historic data) and then use it in nice looking equations and formulas to calculate the efficient frontier and what not.*
I don’t want to dismiss volatility as a risk entirely - it’s e.g. useful to reason why a diversified portfolio will have less variance - but it’s IMO less important than the other two main risk vectors that I see. In fact, I see volalitity more as a chance than as a risk: it allows for picking reasonable entry points in good businesses that are otherwise expensive most of the time.
I would instead categorize risk in these three buckets, in order of importance (blatantly stolen from a SeekingAlpha article by one of Chuck Carnevale’s associates):
There are three kinds of risks all equity investors face.
- fundamental risk: what can impair cash flow, put the payout in jeopardy, and potentially result in total losses (Buffett’s definition of risk)
- valuation risk: so overpaying for a quality company that even if it grows as expected you might suffer years or decades of weak or even negative returns (one of Chuck Carnevale’s key risk definitions)
- volatility risk: becoming a forced seller out of financial/emotional reasons even of quality companies bought at reasonable or attractive valuations
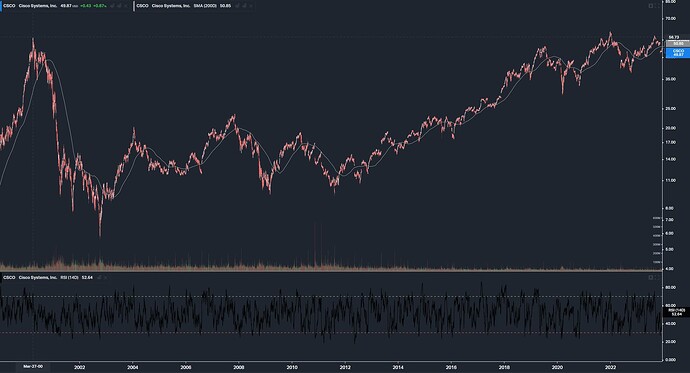
CSCO would be a great example for illustrating “valuation risk”. Buy this great business in March 2000 and then wait 20+ years until the share price is back at when you bought it.
(to be fair, you’d have collected some dividends from CSCO since they initiated one in 2011, but your total return would have been abysmally negative for a good two decades).
You can come up with examples for category “fundamental risk” by yourself (ranging from whale oil producers to horse carriage producers to say … Tesla … hm, ok, let’s pick something less controversial, say, instead … GameStop? Or AMC?).
At any rate, I think you can intuitively understand the risk of “potential total losses”.
And we’ve already discussed the volatility risk. Which IMO is more of a chance, unless your investment approach is wreckless (no safety fund/cash cushion).
Ok, I’m mostly done. Except …
I think there was also a reference to the Efficient Market Hypothesis (EMH) in your initial post, @Julianek, but I’ll glance over that tonight except for stating that it it a hypothesis even as stated by itself.
I would have lots more commentary to add here, but I don’t want to further test your patience. ![]()
* I have further pet peeves with how math is used in MPT (and many other finance “math” pieces) like e.g. the use of variance (or standard deviation) instead of mean absolute deviation. Sure, they’re related, but the former tends to overvalue larger deviations (because of the squaring of differences in the variance). The choice boils down to using the simpler one to calculate rather than the more accurate one.
Maybe using the simpler one (calculation wise) made sense at the time MPT was conceived because of computational constraints, but nowadays when my iPhone (SE) is more powerful than maybe all the compute power at the time the MPT was modelled? C’mon …