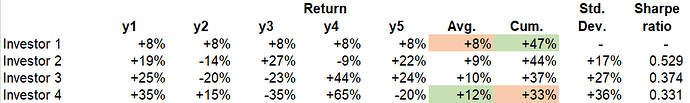Hedging the sequence of return risk

Investing / Portfolios
1

I have recently fixed my collar option strategy (long collar, with e.g. the underlying at 100 you buy a put at 95 and sell a call at 105; the synthetic equivalent would be a bull vertical spread), to roughly lock-in my current +20% YTD return on my main portfolio. Admittedly this is largely market timing looking at the European industry, the upcoming US election and the potential AI bubble and hence not something to recommend. However, it is also an attempt at reducing the volatility of my portfolio, something I have done before very occasionally but never systematically or with an actual logic.

Having recently discussed the sequence of return risk (SRR), I mentioned that I use my own approach of a yield shield strategy (aiming for an FI portfolio of which the passive income covers a lower lean FI target). Alternatively, there is also the standard equity glidepath strategy where you retire with at least a temporary higher bond allocation. The later doesn’t work for me because I don’t link being FI with triggering RE, and don’t want to miss out on the higher expected equity returns long-term.

But now I wonder: Why not systematically hedge portfolio volatility and hence reduce the SRR?

Theory teaches us that volatility is just as important as returns. As an example:

PF Volatility
PF Volatility1016Ă—153 17.7 KB

These are all quite bad portfolios with a Sharpe-ratio (avg. return/std. deviation of returns) below 1, but it shows an example of an inverse correlation between annual average return and cumulative return. The best portfolio is the steady +8% annual of investor 1, the worst the high flying +12% annual of investor 4 because the severe drawdowns kill the cumulative performance.

The contra argument is simple: Hedging is usually not free (a put certainly isn’t and even though a collar option strategy can be free, it still creates opportunity cost long-term), and would inevitably reduce the expected annual return. You would also need to have a somewhat sophisticated understanding of the characteristics of your portfolio to engage in proper hedging, which is not typically given (i.e. it may be a lot of work).

What I am considering is to define a collar option strategy to follow continuously. Something like a simple rule where at X% performance you hedge Y% of your portfolio (e.g. at rolling 12-month performance one standard deviation above long-term expected return you hedge 50% of the portfolio).

Is anyone doing something like that or has an opinion on this? Specifically, I wonder:

  • Would you do this on a continuous 12 month rolling basis? Because obviously there is no argument for a YTD approach.
  • How would it work on the downside? With nice unrealized returns it feels quite simple, but logically you would also hedge in a loss scenario (though maybe not as collar which cuts the upside).
  • How could one approach this to define what levels make sense to hedge and define such a rule? So far, I am acting purely on gut feeling.
2

Maybe you can reduce costs by hedging only the amount you would realise in, say, the next 10-15 years. But I don’t know if there are even products to do this, or if you can do it my continually rolling.

3

Even though it’s very technical for me. I have a question

Let’s say investor has 50,000 USD worth VT shares and they bought them on 1 Jan 2024. What would be the cost to implement the collar option strategy to lock in the gains for next two months?

And what would be maximum loss and maximum profit if stock goes more than 5% up in next two months?

4

VT is easy because you can trade options directly on the ETF, you can just check the pricing Monday once the exchange is online (looks a bit odd at 2am on a weekend). For many other ETFs, like VWRL, you need a proxy (either VT or an index).

For a collar you buy a put and sell a call (aiming to offset the cost of the put). VT is currently at ~120 USD, if you want a 5% collar you would want to buy a put at a strike of 114 and sell a call at 126 (though only 125 is available).

Contract size is 100, so each option represents 12’000 USD. In your example you do it with 4 contracts to represent an underlying of 48’000 USD.

Current ask for a 114 November put is 1.15, or 460 USD total. Current bid for a 125 November put is 0.40, or 160 USD total. With this pricing indication the offsetting of cost doesn’t work and you are out of pocket 300 USD plus fees.

Now if VT moves between 114 and 125 until mid November nothing happens and you lost 300 USD. If VT drops below 114 you can exercise your put and will be covered (getting paid anything below), if VT goes above 125 (even temporarily, these are American style) you must expect to pay out the difference above that (unlimited risk, but of course you gain the same amount on the VT you already own).

1 Like
5

Isn’t that exactly what you are doing? In your example above, you limit your downside to -6.75 per share, but also curtail your upside at 4.25. If markets stay flat, you lose -0.75 (+/- the change in share price).

Am I missing something, or could you achieve the same result by just selling shares and keep cash, bonds, etc.?

6

Thanks for the explanation.
It’s clear

So the key objective of this strategy is to lock in the profits without selling the stocks. However the same can be achieved by selling VT on Monday and buy in November.

I think this could be interesting for cases where either capital gains tax (when selling shares )gets triggered like in US or some EU countries OR investor wants to avoid being classified as trader (if they do it multiple times buy and sell shares)

Right?

1 Like
7

I’m not so hot on my financial mathematical theory, and don’t wish to derail the conversation, but hoping someone who knows more can chime in:

isn’t there some fundamental theorem stating that in an efficient market there is an optimal tradeoff between expected return and volatility. and that therefore any strategy like this can at best fall on this curve (reducing volatility but at the expense of expected return). the point being that you need to compare this ratio relative to other strategies as it might not be optimal.

I’m wondering how the volatility/return tradeoff looks if you just reduced your exposure to stocks and held cash/bonds instead

(maybe this is too simplistic. e.g. in your personal case could be there is some reason that a -5% drop is acceptable but anything worse than that is catastrophic, which would not be captured in the usual framing of volatility)

8

I think to do this at one moment of time (for whatever reason) is interesting. In that case investor doesn’t care about upside because they are happy with what they go.

But to do this on continuous basis will not really work because you wouldn’t know in advance when are good years and when are bad times. Since market tend to go up over time, this way you can lose a lot of returns.

9

I was playing around with options, until I have abandoned it (just in time).

Let’s try to decompose what you are doing. Buying puts is a classic downside protection. If stocks go up, you have just lost some premium, no big harm done. An insurance costs you something. Then you buy new puts protecting the current level. If you try to be more clever, you buy puts further away in maturity, like 9-12 month away, and roll them every 3 month or so. In this way, you lock more capital in puts, but avoid a sharp drop in time value when they approach maturity.

Selling calls, IMO you basically convert the stocks into fixed income if they go up. You collect the premium for it. Here, you should often sell short term ones and roll them, unless you don’t mind to get assigned. If stocks go down, you lose in their value, but collect the premium. I might think about using this strategy if my portfolio is out of balance with too much stocks and consider it a “conditional selling”.

I don’t see what you want to achieve by putting them together. Unless you want to run a fancy strategy fund, of course. Like others have said, why not just sell some stocks? Personally I have time and I decided that there is no better strategy for me than waiting and “just keep buying”.

Your situation is different, of course. But you can try to think about different time to maturity and how it affects the options price.

P.S. in the same vein, you can think about selling puts on securities as “conditional buying” when your portfolio has too little stocks in a downturn. If it comes up again, you have earned some premium, if it went down even further, you have bought.

In both cases, I am not sure if it is better than just maintaining the target allocation, but it might help you to stay the course by letting your inner animal to go on a wild ride from time to time.

1 Like
11

Correct, selling (part of) the portfolio to buy it back later could achieve the same result (as I said, it is market timing). You can even properly calculate how much to sell exactly (called option replication, which has fallen out of fashion because people realized that their internal assumptions are nearly always wrong). If you truly want to transfer all risk (which options don’t) you could of course also go for a future.

Correct, but as per the initial example: Trading some return expectation for a lower volatility might still yield a better cumulative return long-term.

Irrationally I think too strongly in calendar years, that is why I now locked in some of those YTD profits: This year is done, I am happy with what I got, don’t want to have that screwed up by post US election troubles.

Now to address the main issue I wasn’t clear about apparently: I don’t consider to hedge on an ongoing basis. I consider to hedge consistently in line with a strategy. Maybe to extend the example rule:

At rolling 12-month performance one standard deviation above long-term expected return, you hedge 50% of the portfolio at 95% of today’s value for 3 months. If triggered, you don’t to anything for the next 6 months.

The other point I want to clarify: I don’t suggest this as a general long-term strategy, and I am quite certain this would be disadvantageous. I am thinking about this specifically in light of the current lofty valuations and with reference to the sequence of return risk. This is something that only might make sense to consider instead of (or additionally to) the other mentioned approaches like a yield shield or equity glidepath in the years just prior to FI/FIRE and the years immediately after.

This is as much about human psychology as it is about market theory, this is for sure. Maybe it is just that FI is getting nearer and my loss aversion kicks-in to not loose the milestone again once reached (even though I don’t plan to trigger RE immediately).

As expected, your reactions are summarized as: Stupid idea, don’t do it, keep buying and holding. I guess that is exactly what I would have answered to anyone else asking :+1:

3 Likes
13

Not at all. It’s just you should know what you are doing and what the potential consequences are. And this is exactly what you are trying to figure out, and I am happy to help as much as I can.

The latest stupid idea that I came across in this forum I have straightforwardly labelled as such.

1 Like
14

In my opinion, the risk is not to reach a desired outcome at the time it is wanted due to the broader range of outcomes that comes with higher volatility. We may have reached our number at some point but still fall behind full FI at a later stage due to too deep a drop. That may happen at a time when we’ve changed our mind and wouldn’t mind taking the RE part of FIRE as well.

The other side of the coin is that if we sacrifice too much returns to lower volatility, we run the risk of not reaching our target number early enough either. It’s a matter of balance but at some point, working on lowering volatility can make sense in my opinion.

3 Likes
16

Thanks for further clarity
Since your objective is mainly to limit downside, why are you preferring Option 1 over Option 2 ?

Option 1 -: collar option strategy. The con is that it limits the upside too but in some cases it can be cost neutral
Option 2 -: protective PUT strategy. The CON is that it can never have a zero cost.

Is it because Option 1 is less costly?

17

Yes. The example I have given you on VT above is skewed, a real collar can and does offset the majority of the costs. Only buying puts gets expensive fast.

1 Like
18

I’ve only really skimmed over this topic, so apologies if details were already discussed before.

In essence, given the topic title, I would claim that

  • hedging will reduce your total return (you really just pay insurance premiums)
  • hedging will indeed smoothen your sequence of returns risk
  • specific to @1742 's strategy: selling calls might reduce some of the costs, but it probably depends a lot on your broker?